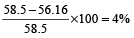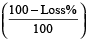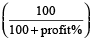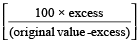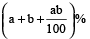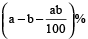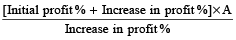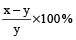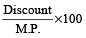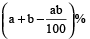Profit, Loss and Discount: Formulas, Tricks, Examples

 Cost Price: The amount paid to purchase an article or the price at which an article is made, is known as its cost price. The cost price is abbreviated as C.P..

Selling Price: The price at which an article is sold, is known as its selling price. The selling price is abbreviated as S.P.

Profit: If the selling price (S.P.) of an article is greater than the cost price (C.P.) , then the difference between the selling price and cost price is called profit.

Thus, If S.P. > C.P., then

Profit = S.P. – C.P.

⇒ S.P. = C.P. + Profit

⇒ C.P. = S.P. – Profit.

Example 1: A shopkeeper buys scientific calculators in bulk for Rs. 15 each. He sells them for Rs. 40 each. Calculate the profit on each calculator in rupees, and as a percentage of the cost price.

Solution: Given: cost price = Rs. 15, selling price = Rs. 40

Profit = selling price – cost price = Rs. 40 – 15 = Rs. 25

the profit as a percentage of the cost price:

Profit % = 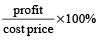profit-loss-f-20089.png

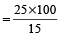profit-loss-f-20098.png% = 166.7%

Loss: If the selling price (S.P.) of an article is less than the cost price (C.P.) , then the difference between the cost price (C.P.) and the selling price (S.P.) is called loss.

Thus, if S.P. < C.P., then Loss = C.P. – S.P. ⇒ C.P. = S.P. + Loss ⇒ S.P. = C.P. – Loss [/av_textblock] [av_textblock size='' av-medium-font-size='' av-small-font-size='' av-mini-font-size='' font_color='' color='' id='' custom_class='ed-text-block-sm-textp' av_uid='av-2wpi6q4' admin_preview_bg=''] Example 2: If the cost price of a book is Rs. 150 and selling price is 137.50, then calculate the loss and percentage loss on the book?

Solution: Here, cost price = Rs. 150

and selling price = Rs. 137.50

∴Loss = Cost price – selling price

= Rs. (150 – 137.50) = Rs. 12.50

Now, Percentage Loss

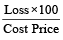= profit-loss-f-20104.png%

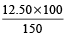= profit-loss-f-20112.png% = 8.33%

Quicker Method to solve the questions

When cost price and percentage profit are given, then selling price

= cost price profit-loss-f-20118.png

Example 3: A chair was purchased for Rs. 470 and sold at a profit of 10%. Find the selling price.

Solution: Using the formula

Selling price = cost price 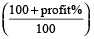profit-loss-f-20124.png

= 470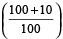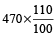profit-loss-f-20134.png= profit-loss-f-20140.png = Rs. 517

When cost price and percentage loss are given, then

Selling price = cost price profit-loss-f-20148.png

Example 4: A person bought a table for Rs. 420 and sold at the loss of 15% . Find the selling price of table?

Solution: Selling price = cost price 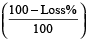profit-loss-f-20154.png

= Rs. 420 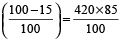profit-loss-f-20164.png

= Rs. 357

When selling price and percentage profit are given, then

Cost price = selling price profit-loss-f-20170.png

Example 5: A Chair was sold for Rs. 517 at a profit of 10%. Find the cost price of the chair.

Solution: Here, selling price = Rs. 517 and profit = 10%

∴ Cost price = selling price 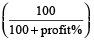profit-loss-f-20179.png

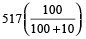
= profit-loss-f-20185.png

= 517 × 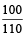profit-loss-f-20191.png= Rs. 470

When selling price and percentage loss are given, then

Cost price = selling price profit-loss-f-20201.png

Example 6: Ram sold a watch for Rs. 376 at a loss of 6%. Find the cost price of the watch.

Solution:

Cost price = selling price 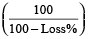profit-loss-f-20207.png

= Rs. 376 × 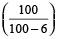profit-loss-f-20215.png

= Rs. 376 × 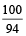profit-loss-f-20221.png= Rs. 400

If two items are sold each at rupees R, one at a gain of x% and other at a loss of x %, there is always an overall loss given byprofit-loss-f-20229.png% and the value of loss is given by profit-loss-f-20235.png.

In case the cost price of both the items is the same and percentage loss and gain are equal, then net loss or profit is zero. The difference between the two cases is that the cost price in the first case is not the same, and in the second case it is the same.

Example 7: Ram sells two Mobile phones for Rs. 1000 each, one at a profit of 10% and other at a loss of 10%. Find his gain or loss percentage.

Solution: Using the formula,

Loss % = profit-loss-f-20243.pngprofit-loss-f-20249.png% = 1%

A dishonest shopkeeper claims to sell goods at cost price, but uses a lighter weight, then his Gain %

= profit-loss-f-20255.png

Example 8: A shopkeeper sells rice to a customer, using false weights and gains 100/8 % on his cost. What weight has he substituted for a kilogram?

Solution: Using the formula,

Gain % = 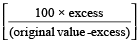profit-loss-f-20267.png

profit-loss-f-20274.png

From here, Excess = 0.111.. Kg, which is 111.11 grams

Weight used by shopkeeper = 1000 – 111.11 = 888.89 grams

Formulas to Remember

Profit = 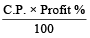profit-loss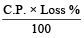-f-20283.png

Loss = p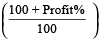rofit-loss-f-20292.png

S.P. = profit-loss-f-20298.png× C.P.

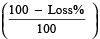S.P. = profit-loss-f-20304.png× C.P.

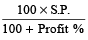
C.P. = profit-loss-f-20310.png

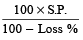
C.P. = profit-loss-f-20319.png

Goods passing through successive hands

When there are two successive profits of a% and b%, then the resultant profit per cent is given by

profit-loss-f-20325.png

When there is a profit of a% and loss by b% in a transaction, then the resultant profit or loss per cent is given by profit-loss-f-20336.png

according to the +ve or –ve sign respectively.

When cost price and selling price are reduced by the same amount (A) and profit increases then cost price (C.P.)

= profit-loss-f-20342.png

Example 9: A table is sold at a profit of 20%. If the cost price and selling price are Rs. 200 less, the profit would be 8% more. Find the cost price.

Solution: By direct method,

C.P. = 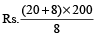profit-loss-f-20348.png

= Rs. 28 × 25 = Rs. 700.

If cost price of x articles is equal to the selling price of y articles, then profit/loss percentage

= profit-loss-f-20355.png

according to +ve or –ve sign respectively.

Example 10: If the C.P. of 15 tables be equal to the S.P. of 20 tables, find the loss per cent.

Solution: By direct method,

Profit/Loss % = 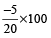profit-loss-f-20361.png

= –25% loss, since it is –ve.

Discount

The reduction made on the ‘marked price’ of an article is called the discount. When no discount is given, ‘selling price’ is the same as ‘marked price’.

Discount = Marked price × Rate of discount.

S.P. = M.P. – Discount.

Discount % = profit-loss-f-20368.png

Example 11: How much % must be added to the cost price of goods so that a profit of 20% must be made after throwing off a discount of 10% from the marked price?

Solution: (c) Let C.P. = Rs. 100, then S.P. = Rs. 120

Also, Let marked price be Rs. x. Then, 90% of x = 120

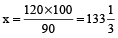
⇒ profit-loss-f-20478.png

∴ M.P. should be Rs. 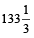profit-loss-f-20472.png

or M.P. = profit-loss-f-20466.pngabove C.P.

Buy x get y free i.e., if x + y articles are sold at cost price of x articles, then the percentage discount

= profit-loss-f-20378.png × 100.

Successive Discounts

In successive discounts, first discount is subtracted from the marked price to get net price after the first discount. Taking this price as the new marked price, the second discount is calculated and it is subtracted from it to get net price after the second discount. Continuing in this manner, we finally obtain the net selling price.

In case of successive discounts a% and b%, the effective discount is:

profit-loss-f-20412.png

Example 12: Find the single discount equivalent to successive discounts of 15% and 20%.

Solution: By direct formula,

Single discount

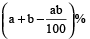
= profit-loss-f-20419.png

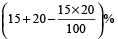
= profit-loss-f-20425.png

= 32 %

If the list price of an item is given and discounts d₁and d₂ are given successively on it then, Final price = list priceprofit-loss-f-20447.png

Example 13: An article is listed at Rs. 65. A customer bought this article for Rs. 56.16 and got two successive discounts of which the first one is 10%. The other rate of discount of this scheme that was allowed by the shopkeeper was :

  1. 3%
  2. 4%
  3. 6%
  4. 2%

Solution: (2): Price of the article after first discount = 65 – 6.5 = Rs. 58.5

Therefore, the second discount

= profit-loss-f-20453.png