Congruence of Triangles - Worksheets
CBSE Worksheet-1
CLASS –VII Mathematics (Congruence of Triangles)
Choose correct option in questions 1 to 4.
- ΔABC and ΔPQR are congruent under the correspondence ABC ↔ RQP
Write the parts of ΔABC that correspond to RQ.
a. AB
b. BC
c. AC
d. none of these - Which angle is included between the sides DE and EF of ΔDEF?
a. ∠D
b. ∠E
c. ∠F
d. none of these - By applying SAS congruence rule, you want to establish that ΔPQR ≅ ΔFED. It is given that PQ = FE and RP = DF. What additional information is needed to establish the congruence?
a. ∠P = ∠D
b. ∠Q = ∠D
c. ∠P = ∠F
d. ∠R = ∠F - Which congruence criterion do you use in the following?
Given: AC = DF, AB = DE, BC = EF. So, ΔABC ≅ ΔDEF
a. ASA rule
b. SAS rule
c. RHS rule
d. SSS rule
Fill in the blanks:
- If two-line segments have the ____________ length, they are congruent.
- If two triangles are congruent, then their ____________ parts (i.e., angles and sides) that match one another are equal.
- In an isosceles triangle base angles opposite to the equal sides are __________.
- The side opposite to the right angle is called the _________ of the right-angled triangle.
- In triangles ABC and PQR, AB = 3.5 cm, BC = 7.1 cm, AC = 5 cm, PQ = 7.1 cm, QR = 5 cm and PR = 3.5 cm. Examine whether the two triangles are congruent or not. If yes, write the congruence relation in symbolic form.
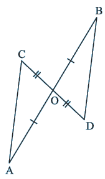
- In the following figure, AB and CD bisect each other at O. State the three pairs of equal parts in two triangles AOC and BOD.
CBSE Worksheet-1
CLASS –VII Mathematics (Congruence of Triangles)
Answer key
- c
Explanation: Since ABC ↔RQP is the correspondence of triangles ΔABC and ΔPQR,
We can say that AB ↔RQ - b
Explanation: The vertex common to the sides DE and EF is E. Hence the included angle is ∠E. - c
Explanation: By SAS congruence rule, two triangles are congruent if two sides and the angle included between them of a triangle are equal to two corresponding sides and the angle included between them of another triangle.
Here Given that PQ = FE and RP = DF, the angle included these two sides are ∠P and ∠F.
Hence ∠P = ∠F. - d
Explanation: Since the three sides of the one triangle is equal to the corresponding sides of the other triangle, as per the SSS congruence criterion is used here. - equal
- corresponding
- equal
- hypotenuse
- Yes ΔABC ≅ ΔRPQ by SSS Congruency
Explanation: The sides of the triangle ABC are AB, BC, AC and that of triangle PQR are PQ, QR, PR
Given that, AB = PR = RP = 3.5cm
BC = PQ = 7.1cm
AC = RQ = 5cm
Hence by SSS congruency rule, since the three sides are equal the triangles ABC and RPQ are congruent
ΔABC ≅ ΔRPQ. - AO = BO, OC = OD and ∠AOC =∠BOD (vertically opposite angle)
Explanation:
Since AB and CD bisect each other at O, AO = BO and OC = OD. Since ∠ AOC and ∠ BOD are vertically opposite angles formed by the intersection of the line segments AB and CD,
∠AOC = ∠BOD
Hence equal parts of the two triangles AOC and BOD are
AO = BO, OC = OD and ∠AOC =∠BOD.
