Mensuration - Solutions 4
CBSE Class –VIII Mathematics
NCERT Solutions
CHAPTER - 11
Mensuration (Ex. 11.4)
NCERT Solutions
CHAPTER - 11
Mensuration (Ex. 11.4)
1. Given a cylindrical tank, in which situation will you find surface area and in which situation volume.
(a) To find how much it can hold.
(b) Number of cement bags required to plaster it.
(c) To find the number of smaller tanks that can be filled with water from it.
(b) Number of cement bags required to plaster it.
(c) To find the number of smaller tanks that can be filled with water from it.
Ans. (a) Volume (it is measure of the amount of space inside of a solild figures)
(b) Surface area (the outside part or uppermost layer of the soild figures)
(c) Volume
2. Diameter of cylinder A is 7 cm and the height is 14 cm. Diameter of cylinder B is 14 cm and height is 7 cm. Without doing any calculations can you suggest whose volume is greater? Verify it by finding the volume of both the cylinders. Check whether the cylinder with greater volume also has greater surface area.
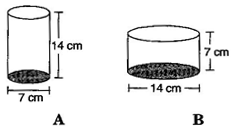
Ans. Yes, we can say that volume of cylinder B is greater, Because radius of cylinder B is greater than that of cylinder A.

Ans. Yes, we can say that volume of cylinder B is greater, Because radius of cylinder B is greater than that of cylinder A.
Diameter of cylinder A = 7 cm
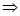 Radius(r) of cylinder A =
Radius(r) of cylinder A =  cm and Height(h) of cylinder A = 14 cm
cm and Height(h) of cylinder A = 14 cm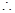 Volume of cylinder A =
Volume of cylinder A = 
= 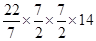
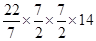
= 539 

Now Diameter of cylinder B = 14 cm
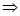 Radius of cylinder B =
Radius of cylinder B = 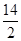 = 7 cm and Height of cylinder B = 7 cm
= 7 cm and Height of cylinder B = 7 cm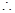 Volume of cylinder A =
Volume of cylinder A = 
=  cm3
cm3
 cm3
cm3
= 1078 

Since the cylinder A and cylinder B is open from upper end then it will exclude from the Total surface area
Total surface area of cylinder A = ( Area of lower end circle + curved surface area of cyliner)
=( + )
=
= x (+2x14)
= 11 (+28)
= 11(31.5) cm2 = 346.5 cm2
Total surface area of cylinder B = 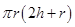
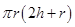
= x 7(2x7+7)
= 22  (14 + 7)
(14 + 7)
 (14 + 7)
(14 + 7)
= 22  21 = 462
21 = 462
 21 = 462
21 = 462
Yes, cylinder with greater volume also has greater surface area.
3. Find the height of a cuboid whose base area is 180  and volume is 900
and volume is 900 ?
?
 and volume is 900
and volume is 900 ?
?
Ans. Let the Length, breadth and height of the cuboid be l, b, h.
Base of the cuboid is form a Recatangle so,that the Base(Reactangle) Area is (Length x Breadth)
Base area of cuboid = 180 

L x B = 180 cm2 .......................................(1)
Volume of cuboid = 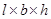
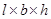
Volume of cuboid = 900 

= 900 (From eq. 1)
(180) h = 900

= 5 m
Hence the height of cuboid is 5 m.
4. A cuboid is of dimensions 60 cm  54 cm
54 cm  30 cm. How many small cubes with side 6 cm can be placed in the given cuboid?
30 cm. How many small cubes with side 6 cm can be placed in the given cuboid?
 54 cm
54 cm  30 cm. How many small cubes with side 6 cm can be placed in the given cuboid?
30 cm. How many small cubes with side 6 cm can be placed in the given cuboid?
Ans. Given: Length of cuboid 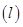 = 60 cm,
= 60 cm,
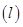 = 60 cm,
= 60 cm,
Breadth of cuboid 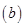 = 54 cm and
= 54 cm and
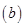 = 54 cm and
= 54 cm and
Height of cuboid 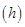 = 30 cm
= 30 cm
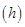 = 30 cm
= 30 cm
We know that, Volume of cuboid = 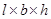
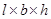
= (60  54
54  30)
30) 
 54
54  30)
30) 
And Volume of cube = (Side)3
= 6  6
6  6
6 
 6
6  6
6 
 Number of small cubes =
Number of small cubes = 
= 450
Hence required number of small cubes are 450.
5. Find the height of the cylinder whose volume if 1.54  and diameter of the base is 140 cm.
and diameter of the base is 140 cm.
 and diameter of the base is 140 cm.
and diameter of the base is 140 cm.
Ans. Given: Volume of cylinder = 1.54 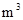 and Diameter of cylinder = 140 cm
and Diameter of cylinder = 140 cm
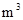 and Diameter of cylinder = 140 cm
and Diameter of cylinder = 140 cm Radius
Radius 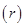 =
= 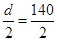 = 70 cm
= 70 cm
= m = 0.7m [
Volume of cylinder = 

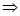 1.54 =
1.54 = 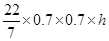
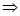
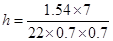
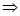
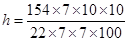
= 1 m
Hence height of the cylinder is 1 m.
6. A milk tank is in the form of cylinder whose radius is 1.5 m and length is 7 m. Find the quantity of milk in liters that can be stored in the tank.
Ans. Given: Radius of cylindrical tank 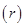 = 1.5 m
= 1.5 m
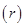 = 1.5 m
= 1.5 m
Height of cylindrical tank  = 7 m
= 7 m
Volume of cylindrical tank =
 = 7 m
= 7 m
Volume of cylindrical tank =

= 

= 49.5 m3
= 49.5  1000 liters [
1000 liters [ 1
1 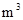 = 1000 liters]
= 1000 liters]
 1000 liters [
1000 liters [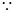 1
1 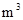 = 1000 liters]
= 1000 liters]
= 49500 liters
Hence required quantity of milk is 49500 liters that can be stored in the tank.
7. If each edge of a cube is doubled,
(i) how many times will its surface area increase?
(ii) how many times will its volume increase?
Ans. Let units be the edge of the cube.
Surface area = and Volume of the cube =
When its edge is doubled =
(i) Surface area =6(side)2
= =
=
= 4 (Surface area)
The surface area of the new cube will be 4 times that of the original cube.
(ii) Volume of cube (V) = 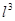
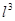
When edge of cube is doubled = , then
Volume of cube (V’)=
V’ = 8(Volume of cube)
Hence volume will increase 8 times.
8. Water is pouring into a cuboidal reservoir at the rate of 60 liters per minute. If the volume of reservoir is 108 , find the number of hours it will take to fill the reservoir.
, find the number of hours it will take to fill the reservoir.
 , find the number of hours it will take to fill the reservoir.
, find the number of hours it will take to fill the reservoir.
Ans. Volume of reservoir = 108 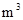
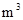
= 108 x 1000 litres
=108000 litres
Since water is pouring into reservoir @ 60 litres per minute and in
Time taken to fill the reservoir = x hours
= 30 hours
Hence, 30 hours it will take to fill the reservoir.
